CalculuS
Learn smarter, practice harder. Skill-building made simple, fun, and effective. Mprezz turns effort into expertise
Vector Integration
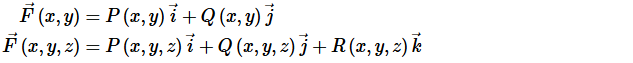
Conservative field
A vector field for which there exists a scalar function f such that ∇f=F.
Gradient field
A vector field F for which there exists a scalar function f such that ∇f=F in other words, a vector field that is the gradient of a function; such vector fields are also called conservative.
Potential function
A scalar function f such that ∇f=F.
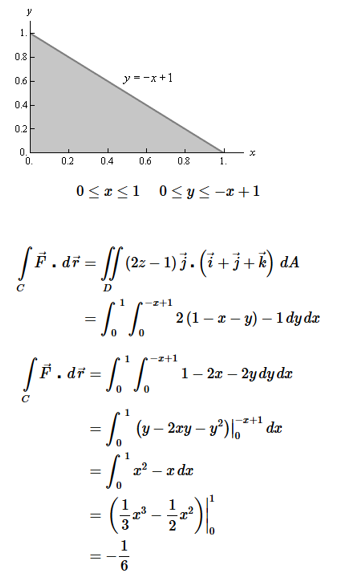
Line Integral
1.

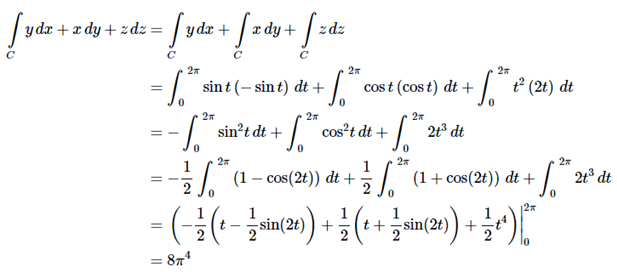
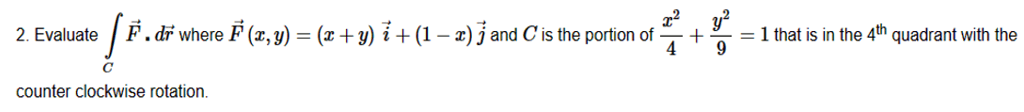
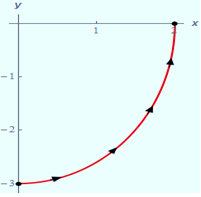
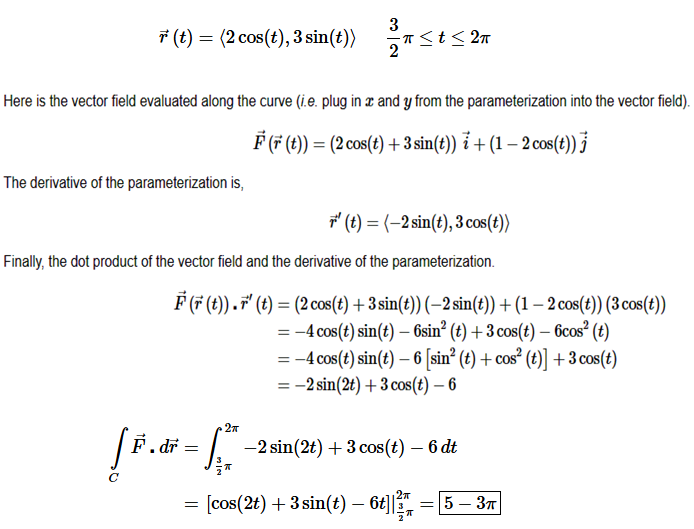

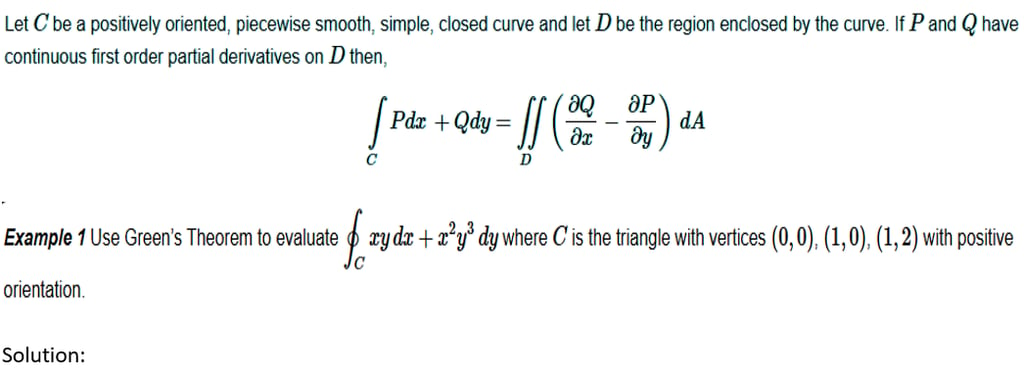
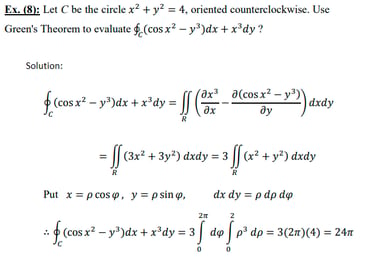
Green’s Theorem

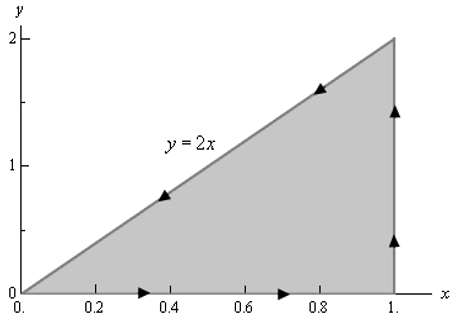


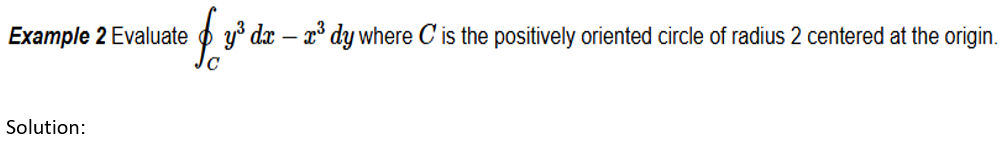
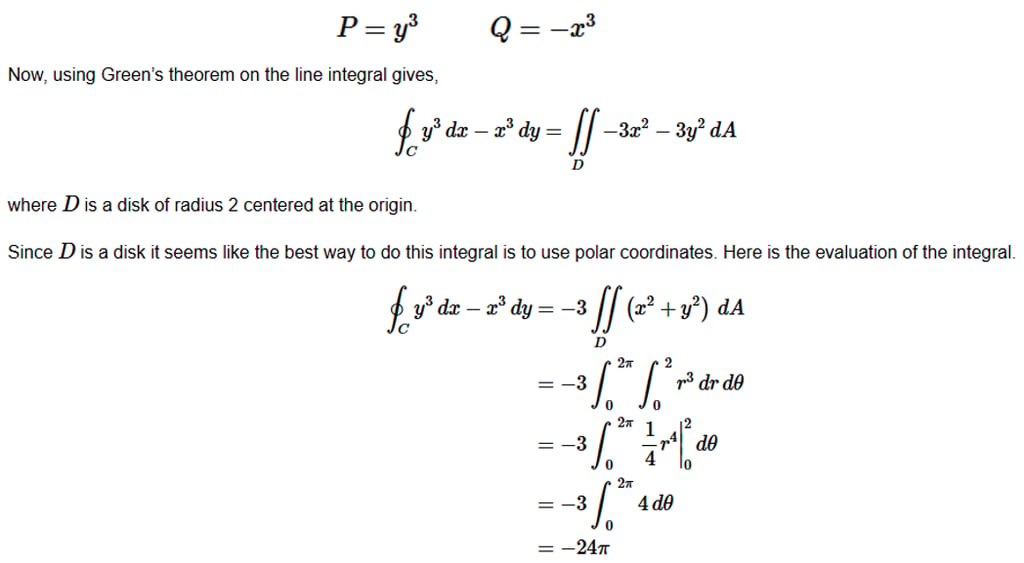

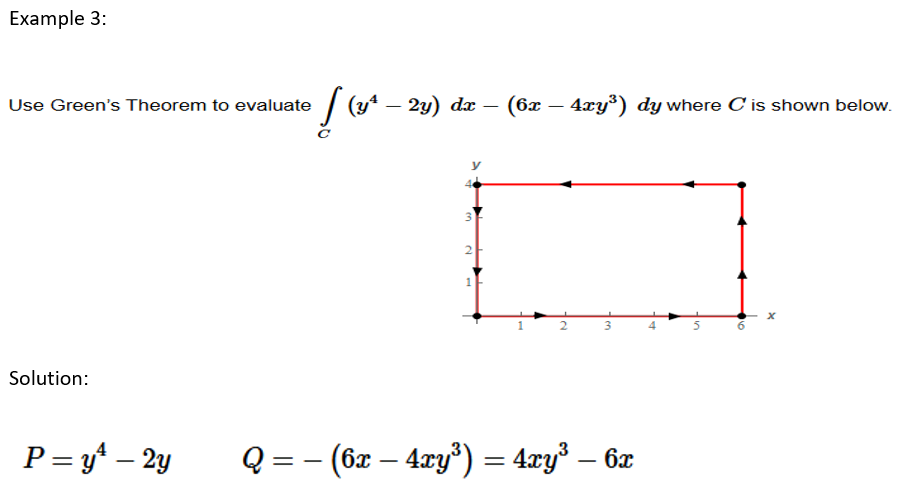
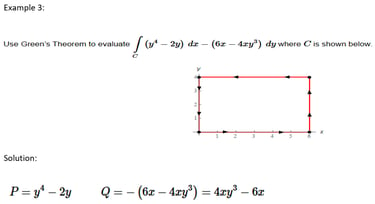
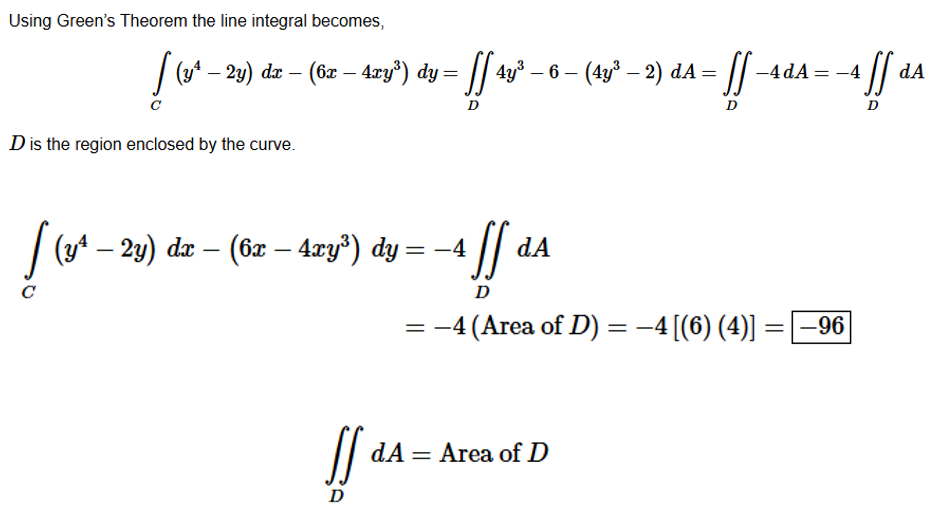
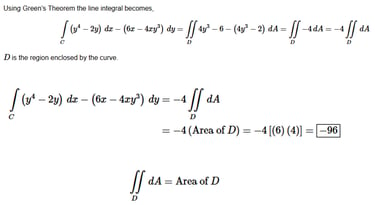


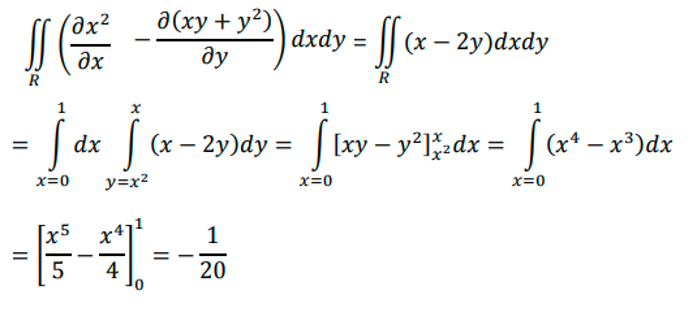
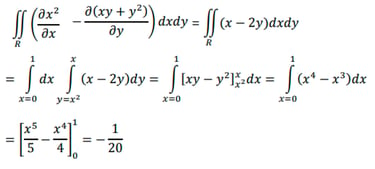

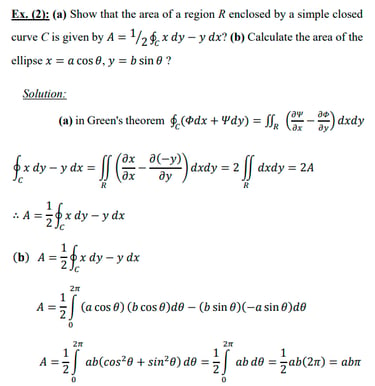
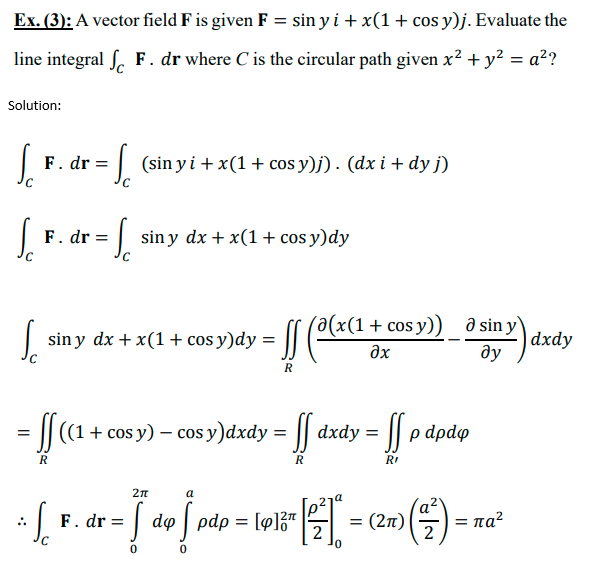
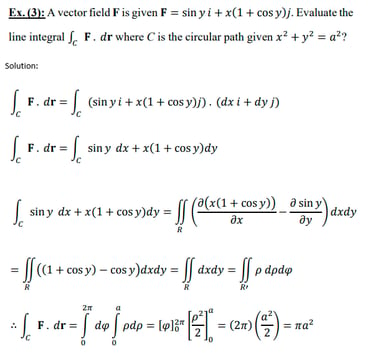
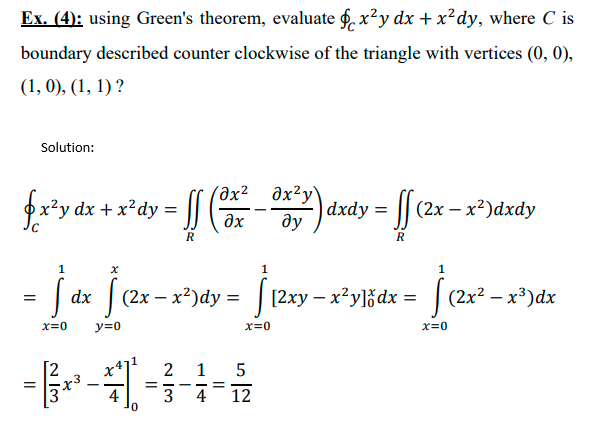
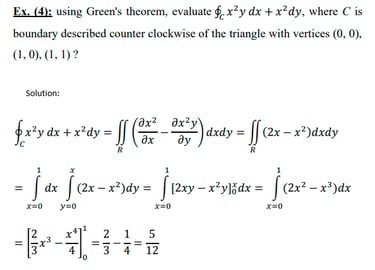
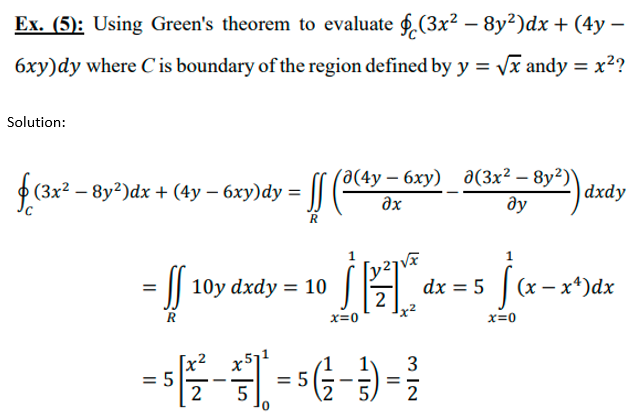
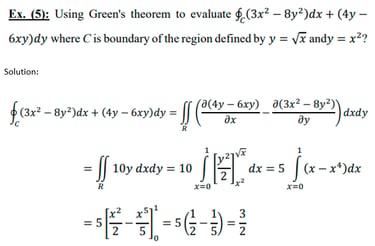
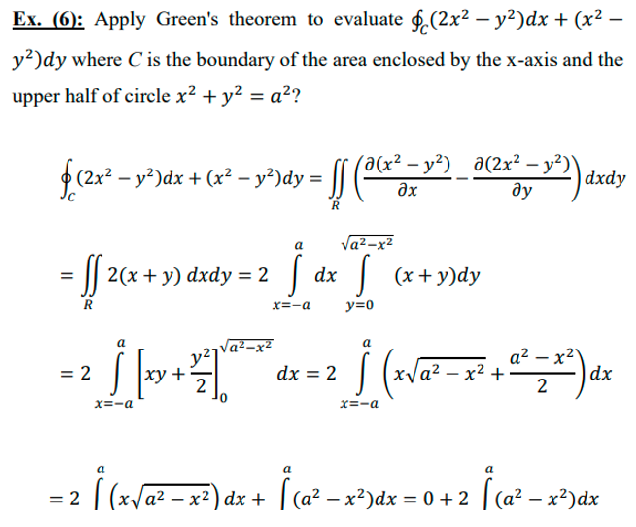
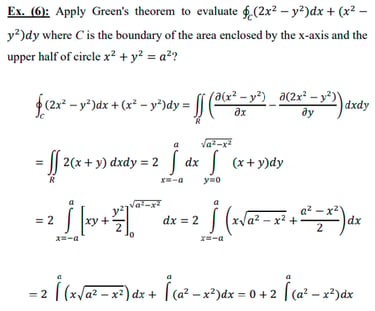
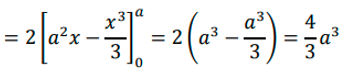
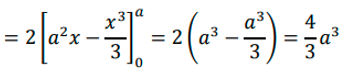
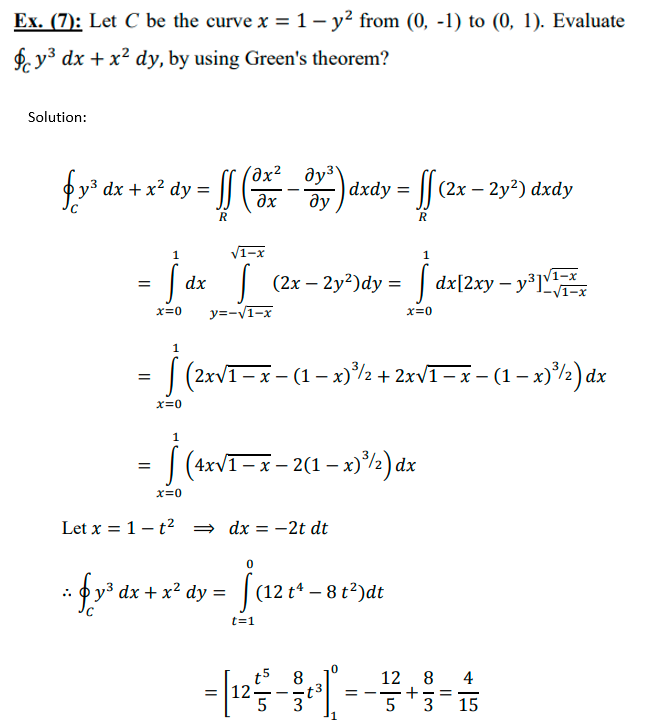
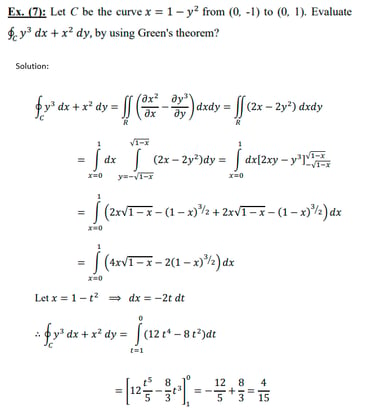
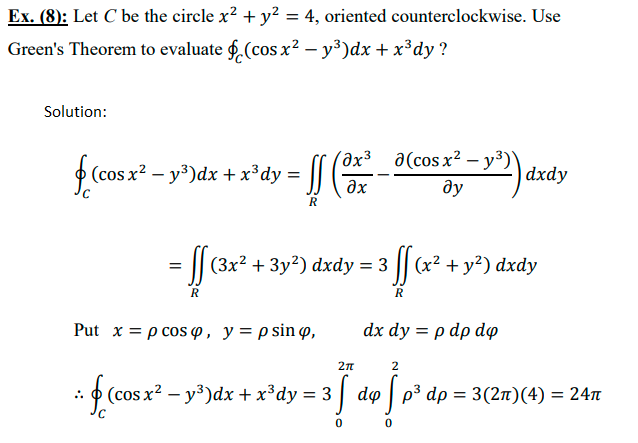

Gauss Divergence Theorem
Let E be a simple solid region and S is the boundary surface of E with positive orientation. Let F be a vector field whose components have continuous first order partial derivatives. Then,
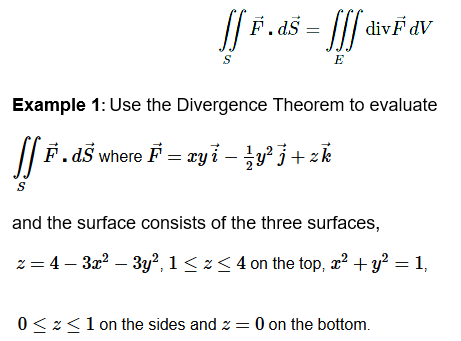
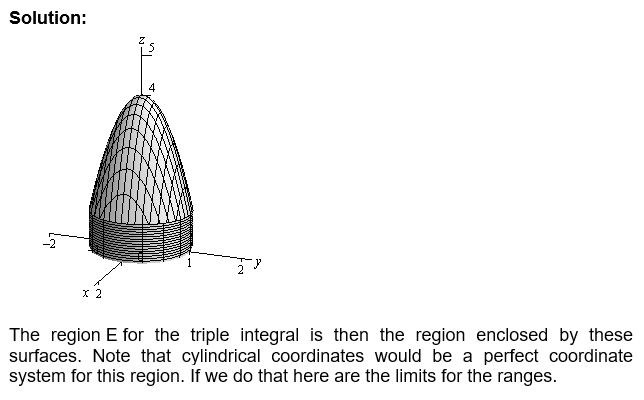
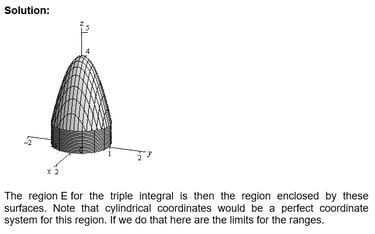
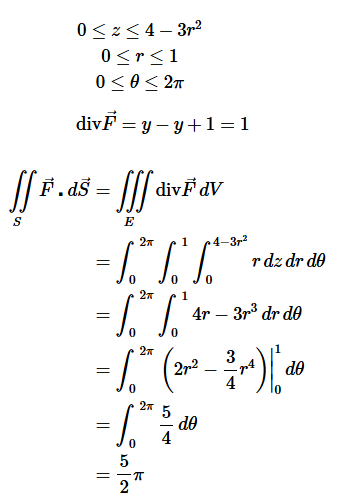
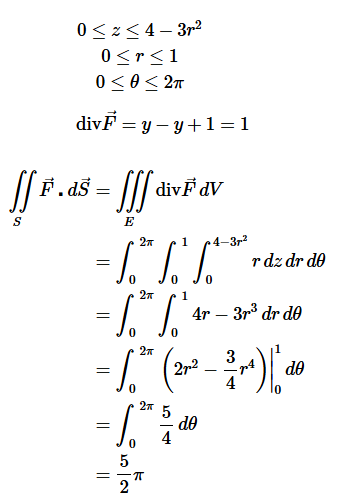
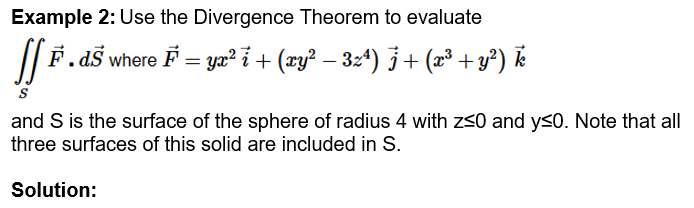

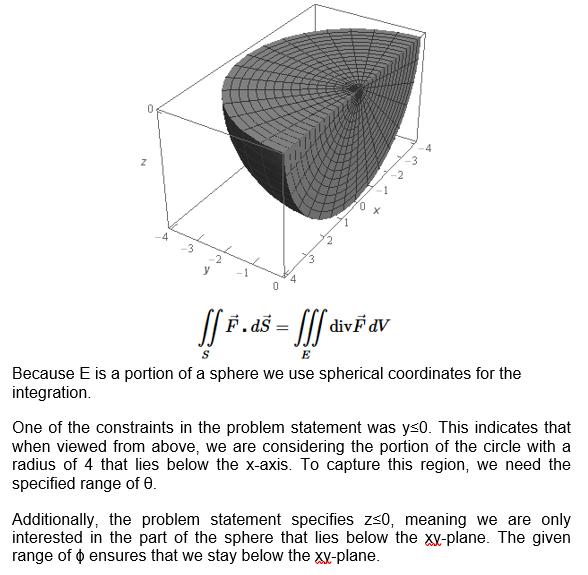
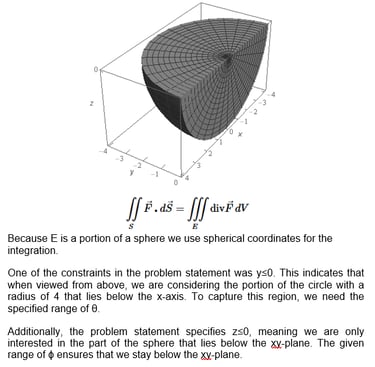
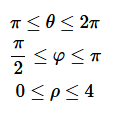
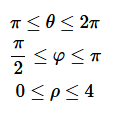
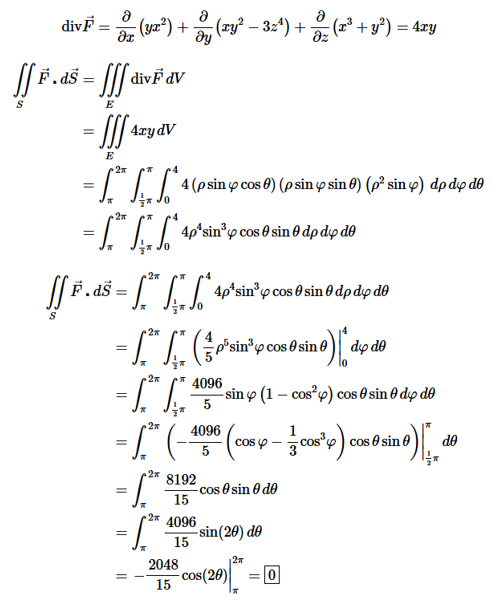

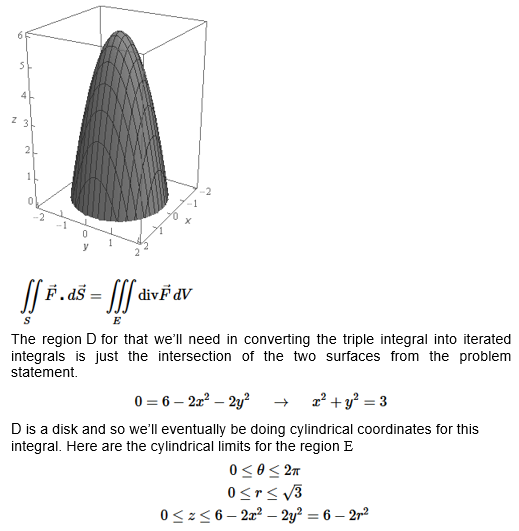
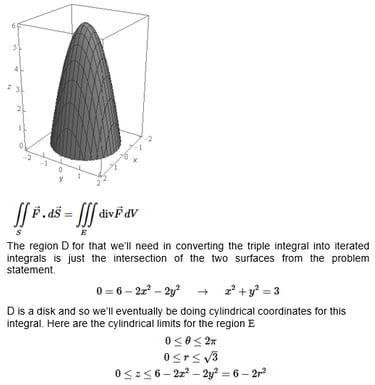
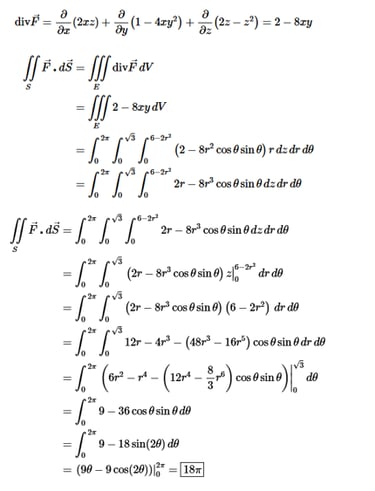
Example 3: Use the Divergence Theorem to evaluate
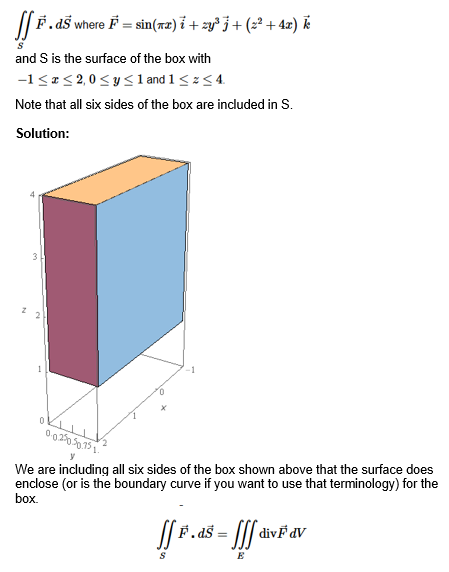
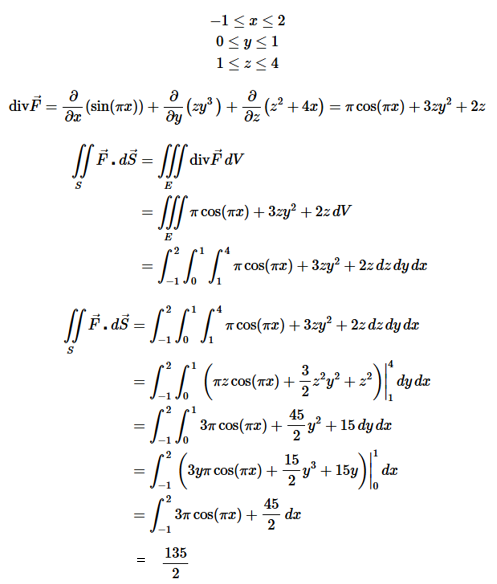
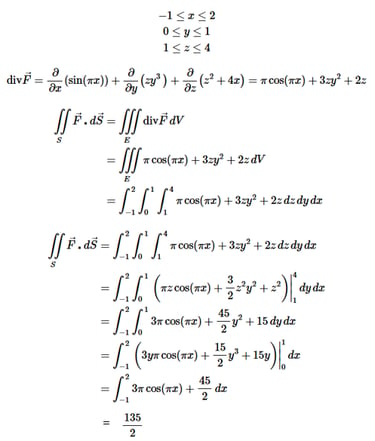


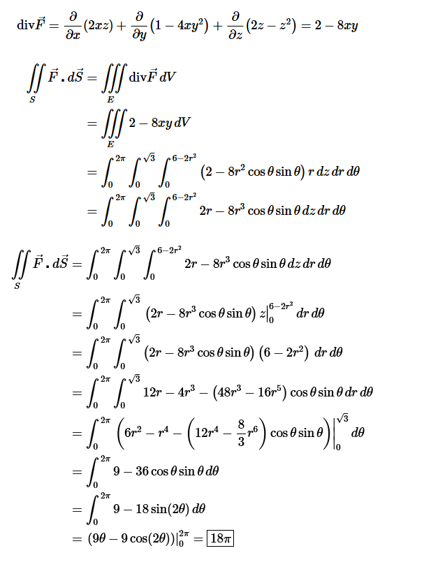
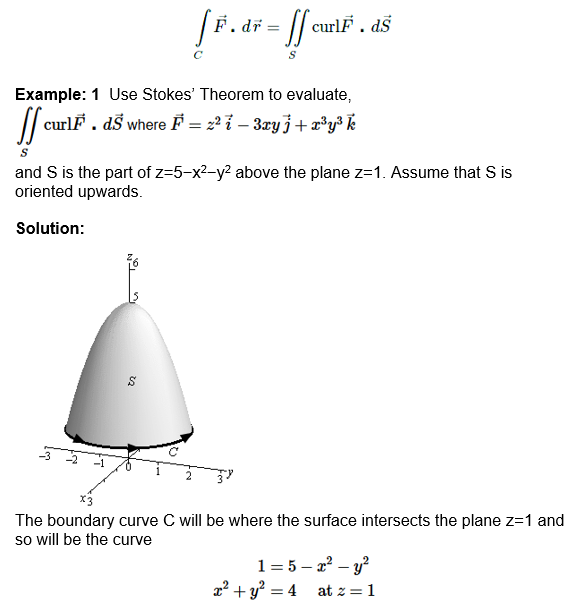
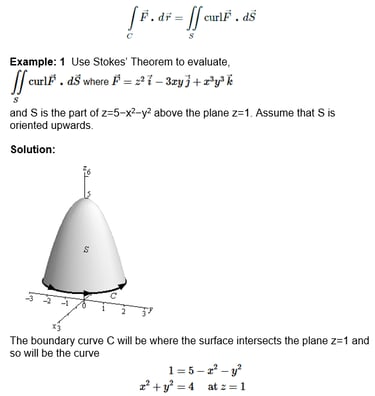
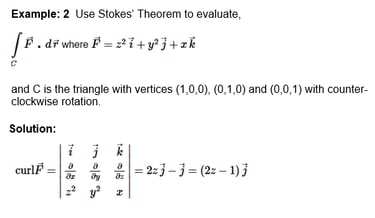
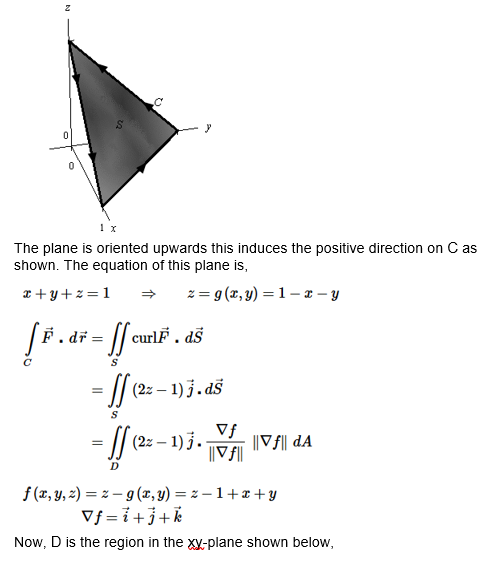
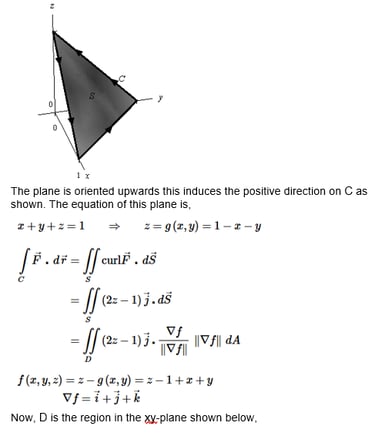
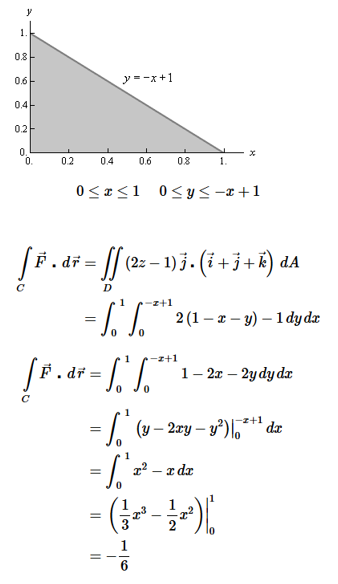
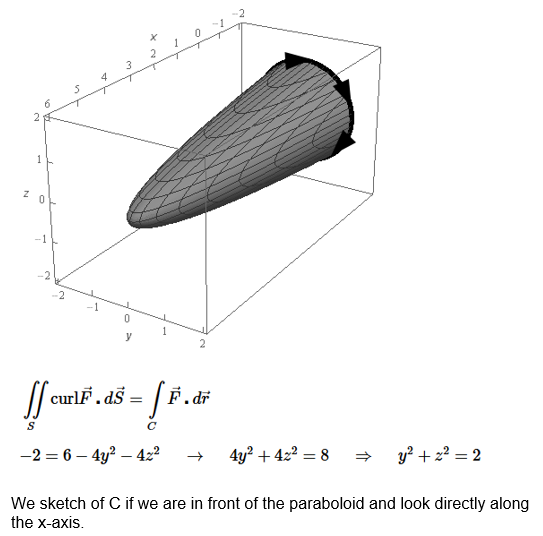
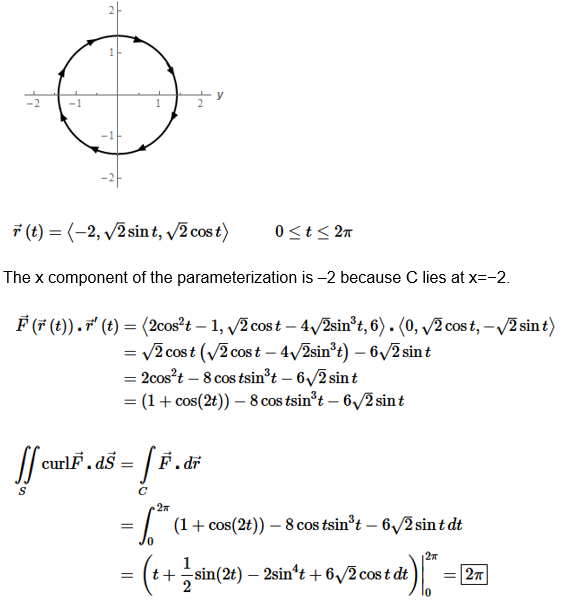
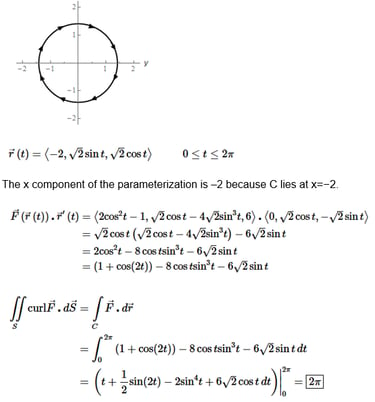
Stoke’s Theorem




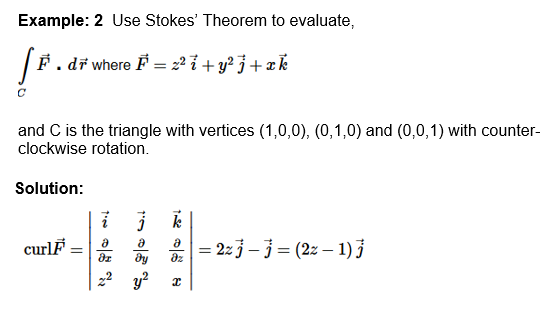


© 2024. All rights reserved.
Professor of Practice
Bridging academia and industry through innovative teaching and real-world experience for academic success.
